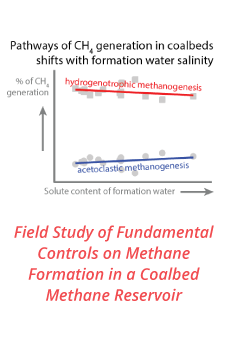
Reports: DNI954427-DNI9: A Fundamental Study on Efficient Resolution of the Inherent Nonlinearity of Compositional Formulations for Flow in Porous Media
Mohammad Shahvali, PhD, University of Tulsa
This project has aimed to find a viable, efficient and robust nonlinear solution strategy for multiphase flow in porous media. To serve this purpose, two fundamental hypotheses with regard to the nonlinear coupling between the equations are proposed: Firstly, we hypothesize that the pressure variables can be separated and updated independently from the saturation variables. Secondly, we hypothesize that the system of hyperbolic conservation equations can be entirely decoupled to solve. The hypotheses proposed in this project, raise a fundamental question: Can we have a numerically stable and convergent nonlinear solution strategy based on separation of pressure and saturation variables? To verify the hypotheses, we proposed a new nonlinear solution strategy that is applicable to the conventional Fully Implicit discretization of the governing equations of the multiphase flow and transport in porous media. The traditional Newton-Raphson solver applied to the fully coupled system of equations has a major drawback:
The convergence of the nonlinear iterations is often poor as a result of the increasingly unbearable degree of nonlinear coupling, especially when flow reversal over Newton iterations occurs frequently. Even when convergence is obtained, the computational cost for solving the full system of equations at each Newton iteration is too high. Conventional sequential-implicit strategies can reduce computation cost during each nonlinear iteration, but they suffer from severe restrictions on the allowable timestep size. Our framework concurrently addresses the problems of convergence and computational cost per each nonlinear iteration. On one hand, we decouple the pressure and saturation updates via a two-step scheme comprised of the linear pressure update in a reduced system, followed by the saturation update step. On the other hand we address the flow reversal phenomenon by treating the counter-current and co-current flow regions differently.
Our results show that the proposed algorithm allows for larger timestep sizes during the simulation timeframe. Moreover, as compared to the standard Newton solver, the computational cost associated with each nonlinear iteration is reduced significantly as a result of smaller linear systems of equations that need to be solved.
In addition, the total number of nonlinear iterations are comparable to the state-of-the-art solvers. Despite the advantages of the proposed framework, a workable implementation of the solver is not straightforward. We demonstrate that the choice of linear solver is critical to implement a simulator based on the proposed nonlinear solver. We pinpoint numerous potential pitfalls when implementing the proposed framework to outperform Newton-Raphson method using modern high level programming languages. Ultimately our algorithmic analysis and timing results show that the optimized version of the proposed solver is not able to outperform Newton-Raphson method when timestep sizes are small; however, for more practical simulation cases when larger timesteps are selected, the algorithm can outperform the state-of-the-art solvers.
The impact of the research on the PI’s career and the participating student:
This project has had a great impact on the PI’s career trajectory. As part of this project, major collaboration with other faculty members involved in reservoir simulation research took place. In particular, the PI collaborated with a new research consortium at the department and ultimately secured his position as a Co-Principal Investigator. Moreover, the PI had several constructive discussions with industry experts, thereby drawing a great deal of attention to his research endeavors. One Master’s student was assigned to this project. The student had to acquire some technical background both in terms of fundamentals and implementation skills. As a result of working on this project, the student has gained a wide range of skills which he can utilize in future projects or industry positions.