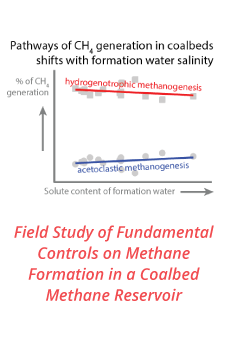
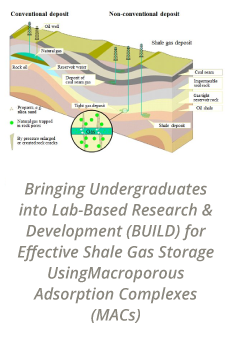
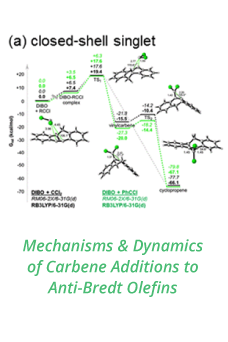
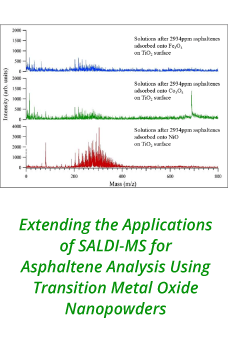
Reports: DNI853314-DNI8: Flow Response to Transient Forcing in Porous Media
Christian Huber, PhD, Georgia Institute of Technology
In this study, we are interested to model, at the pore
scale, the exchange of stresses and energy between a fluid and its porous host
under various conditions. In the first half of our study (last year's report),
with a PhD student Yanqing Su, we focused on the
transmission of dynamical stresses in the pore fluid and the effect of
frequency over the dynamical response of the saturated porous media (Huber and
Su,
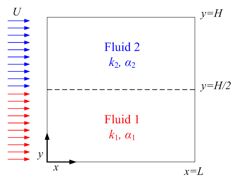
Fig 1. Schematic of 2D convective channel with horizontal
interface, from Karani and Huber (2015)
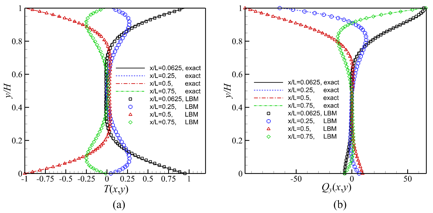
Fig. 2. Comparison of LBM with the analytical solution: (a)
temperature, (b) vertical heat flux, for H=L=1, L/
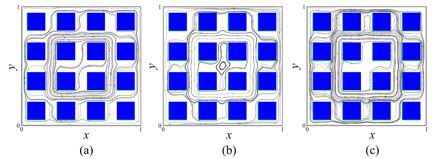
Fig. 3. Streamlines for Ra=105: (a) ks/kf=0.1, (b) ks/kf=10, (c) ks/kf=100 from Karani and Huber (2015).