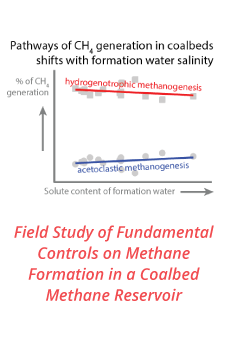
Reports: ND953466-ND9: Molecular Theory and Constitutive Models for Wormlike Micellar Solutions
Michael D. Graham, PhD, University of Wisconsin (Madison)
Dilute solutions of wormlike micelles are important in various applications ranging from fracking fluids to heat transfer media to personal care products. Such solutions exhibit complex rheological behavior such as shear thickening, shear thinning, and formation of gel like structures under flow. While the fundamental physics governing the rheology of dilute micellar solutions has been known for some time, constitutive models relating the extra stress to the imposed strain are not yet available. Without proper constitutive models, higher level simulations (e.g. multiphysics applications) involving wormlike micelles cannot be constructed. In this project, we aim to develop constitutive models at two different levels of abstractions -- (i) models based on direct experimental observations, and (ii) models derived from a suitable microscopic description of micellar solutions. In the end, the hope is that the two kinds of models will be mutually consistent, allowing other researchers in the field to use whichever they deem suitable for their purpose.
As a first step in developing models based on direct experimental observations (phenomenology), we have explored the applicability of a model developed by Bautista and coworkers to capture the behavior of wormlike micellar solutions. Although this model was originally developed for thixoptropic materials, we believe it contains some of the key ingredients necessary to model micellar solutions. A direct application of this model predicts initial shear thickening on imposition of shear/extensional flow, which is also observed experimentally. However, it fails to predict a gelation transition at a finite strain rate. A variant of this model can predict a gelation like behavior, where the viscosity blows up at a finite strain rate, but it cannot account for any rheological behavior past the gel point. While adding enough parameters to the model results in the desired behavior, the exact form of the equations require some educated guesses. We expect some guidance will come from looking at a microscopic picture.
For a microscopic model, we are considering a dilute suspension of rods that can react to grow in length, while undergoing spontaneous scission to break down into smaller rods. Cates and coworkers have shown that a gelation transition exists for such a model. We have coupled the time evolution of the rod length to a kinetic theoretical description of the evolution of the distribution function for the rod. The complexity of these equations are non-trivial, and we are working on methods for solving these equations. Once we have an expression for stress from the microscopic model, we can resume development of our phenomenological model, equipped with a clearer picture of the necessary parameterization.