61st Annual Report on Research 2016

The objective of this project is to quantify nonlinear transport processes in electrolytes under oscillatory voltages. The response of charge-laden media subject to electric fields plays an important role in the petroleum industry: e.g. application of electrical currents to deep lying reservoirs has been claimed to enhance oil recovery. Electrolytes are also subject to time-dependent voltages in electrochemical energy storage and conversion devices (e.g. batteries and super-capacitors); ion channels; synthetic nanopores; directed assembly; and microfluidics. The standard technique to characterize transport processes in an electrolyte is Electrochemical Impedance Spectroscopy (EIS), where a sample is subject to a small amplitude ac voltage. The ratio of voltage to measured current equals the complex, frequency-dependent impedance of the material: the real part (in-phase with voltage) yields the resistance, whose inverse is conductivity, and the imaginary part (out-of-phase) yields capacitance. The capacitance is proportional to the electrical energy stored at an electrode-electrolyte interface, which is an important quantity for super-capacitors. Equivalent circuit models are frequently used to interpret EIS experiments, wherein the micro-scale ion dynamics are replaced by electronic circuit elements. Notably, circuit models assume that the impedance is independent of the magnitude of the applied voltage and constant in time, which requires that the current must vary at the same frequency as the imposed voltage. Practically, this scenario is realized only when the maximum amplitude of the applied voltage V₀ does not exceed the 'thermal voltage' V_T = k_BT/e, where k_B is Boltzmann's constant, T is absolute temperature, and e is the charge on a proton (V_T Å 25mV at T = 300K). However, it is common for applied voltages far exceeding V_T to be applied across electrolytes, particularly in non-polar solvents relevant to the petroleum industry. Here, the electrolyte is driven far from equilibrium, and the concept of impedance as a ratio of voltage over current loses meaning. There is a critical gap in understanding of electrolyte dynamics in this nonlinear regime; traditional (linear) EIS cannot infer the conductivity and capacitance at these conditions. This motivated our project to design a modeling framework to quantify electrolyte dynamics under large-amplitude, transient voltage.

A major accomplishment of our project is the development of a theoretical model to predict the dynamical response of an electrolyte under a moderately nonlinear oscillatory voltage. This fulfilled a central aim of the proposed research effort. This work was published in Physical Review E in 2015. Specifically, we quantified the dynamics of a symmetric binary electrolyte between two blocking electrodes under an ac voltage. The diffuse charge dynamics were modeled via the Poisson-Nernst-Planck (PNP) nonlinear partial differential equations for point-like, non-interacting ions. The solution to these equations was expressed as a Fourier series with an asymptotic expansion of Fourier modes in voltage amplitude (V₀), for arbitrary Debye layer thickness and ac frequency. We demonstrated that the response of the electrolyte remains essentially linear in voltage amplitude at frequencies greater than the RC frequency of Debye layer charging, D/λ_DL, where D is the ion diffusivity, λ_D is the Debye length, and L is half the cell width. In contrast, nonlinear response was predicted at frequencies below the RC frequency. These results were used to compute a generalized voltage-dependent impedance, the first nonlinear contribution to which is quadratic in V₀. This contribution predicts a decrease in the imaginary part of the impedance at low frequency, which is due to the increase in Debye layer capacitance with increasing V₀. In contrast, the real part of the impedance increases at low frequency, due to adsorption of neutral salt from the bulk to the Debye layer. In summary, we demonstrated that a meaningful notion of impedance can be formulated at large voltages, which encodes physical information on the nonlinear electrolyte response.

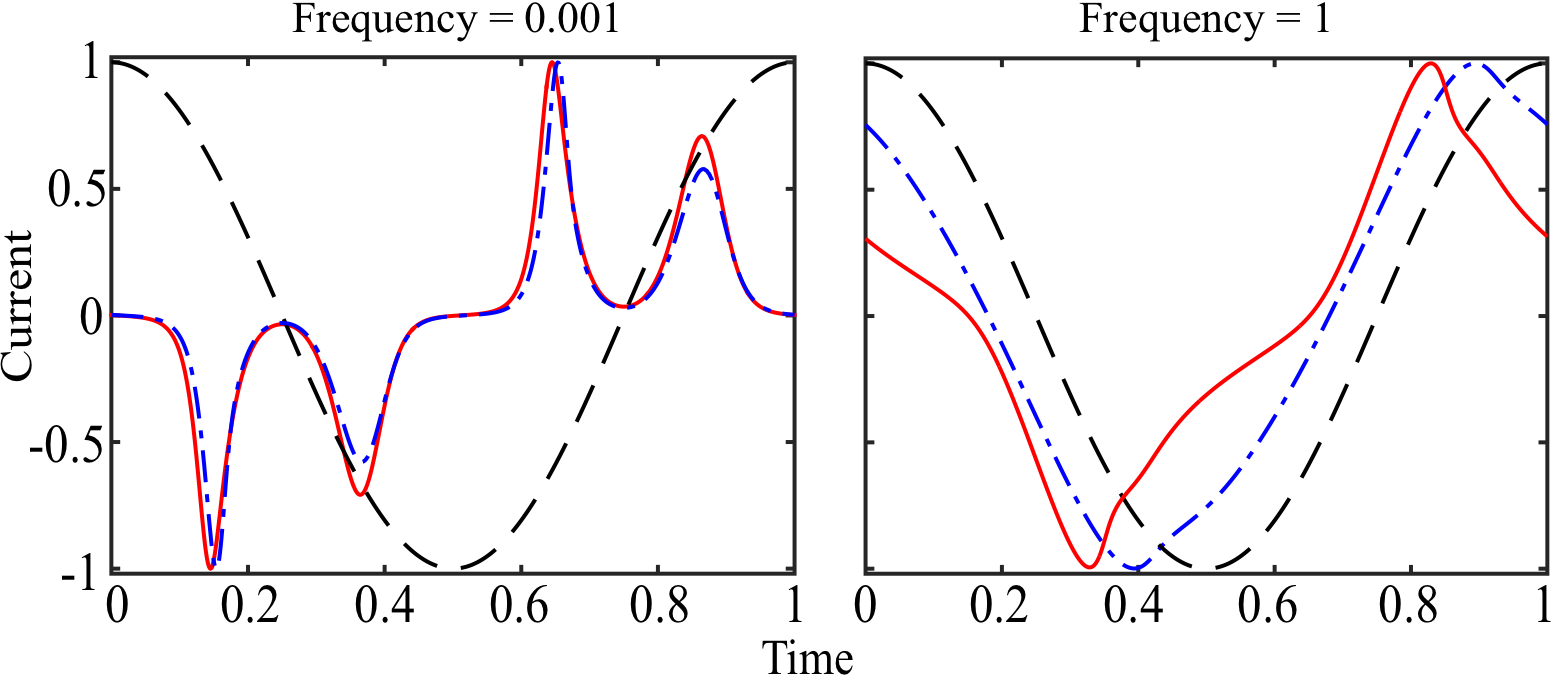
We continue to develop a numerical scheme to solve the PNP equations under strongly nonlinear ac voltages, far exceeding V_T. Of particular interest is to quantify the impact of unequal ion diffusivities on the temporal current evolution. Note that in any real electrolyte system the diffusivities of anions and cations are unequal to some degree. Preliminary results highlight that unequal diffusivities primarily impact the current evolution at frequencies on the "ambipolar" RC time D_a/λ_DL, where D_a is the ambipolar diffusivity: D_a = 2D_pD_m/(D_p+D_m), where D_p and D_m are the diffusion coefficients of the cations and anions, respectively. At lower frequencies, the difference in diffusion coefficients plays a less pronounced role in the current evolution (figure 1). Finally, we aim to validate our theoretical predictions against experimental measurements, which will be performed in collaboration with colleagues at Carnegie Mellon University.

Figure 1: Numerical solution of the PNP equations for nonlinear transient current in response to an oscillating voltage (dashed line) of magnitude V₀/V_T = 15, as a function of dimensionless frequency (normalized by D_a/λ_DL). Dimensionless current (normalized by AeDV_T/ λ_DL², where e is the permittivity and A is the electrode surface area) is plotted over one period of voltage oscillation after initial transients had died out. The cases of equal diffusivities and unequal diffusivities (latter with ratio of five) are shown as dot-dashed and solid lines, respectively. The impact of unequal diffusivities is diminished at low frequencies, where near-equilibrium Debye layers form at opposing electrodes.

The scientific impact of this research includes one journal publication, and two oral presentations: at the ACS Colloid and Surface Science Symposium in June 2015 and AIChE Annual Meeting in November 2015. The research has provided partial support for three graduate students: Robert Stout, Nicholas Chisholm, and Rajarshi Sengupta, who have each received specialist training in electrochemical transport processes and applied mathematics. The project funding has positively impacted the Principal Investigator's (PI's) career by facilitating continuation of his research interests in electrochemical transport processes.