61st Annual Report on Research 2016

High sensitivity piezoelectric materials have been developed as follows. Lattice structures are presented with a piezoelectric sensitivity, d, much larger in magnitude than that of material comprising the lattice ribs. Large sensitivity is achieved by using piezo- electric bimorph elements as ribs; these bend in response to electric input, giving rise to a much larger displacement than for axial elements. Complex electrical connectivity is helpful but not necessary; surface contact can be sufficient. Lattices are amenable to piezoelectric vibration damping. Piezoelectric sensitivity can be arbitrarily large and is unbounded. A sensitive piezoelectric composite lattice was developed and tested by experiment. Lattice structures based on bimorph rib elements are fabricated and studied experimentally. The effective piezoelectric sensitivity d is observed to be much larger, by a factor of at least 10,000, in magnitude than that of material comprising the lattice ribs. Bending of the ribs in response to input voltage is responsible for the large sensitivity.

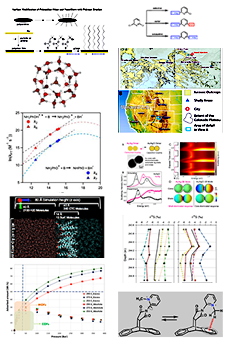
Enhancement of piezoelectric sensitivity and reciprocity failure was obtained in a piezoelectric polymer composite. Reciprocity principles, which entail equivalent outcome on exchange of cause and effect, are widely used and accepted. We present a piezoelectric composite system designed so that reciprocity does not hold; sensitivity is substantially enhanced. Reciprocity failure is observed in which the piezoelectric direct effect (stress causes polarization) sensitivity d is unequal to the converse effect (electric field causes deformation) sensitivity d. The piezoelectric polymer PVDF under isothermal conditions on a polymer substrate obeys reciprocity. Reciprocity failure occurs when a bumpy contact condition causes stress gradients. Reciprocity failure with strong frequency dependence occurs in the presence of thermal flux that is modulated by force: a non-equilibrium condition. Non-reciprocal effects give rise to a maximum enhancement of a factor of five in sensitivity.

In the study of reciprocity, temperature and substrate dependence of piezoelectric sensitivity was observed for PVDF films. The piezoelectric sensitivity, via both the direct and converse effects, for commercial polyvinylidene fluoride (PVDF) films is measured as a function of temperature and frequency, for two substrates, nylon and aluminum. The average effective sensitivity for the PVDF on nylon was 29 pm/V for both direct and converse effect, independent of frequency over 0.5 to 200 Hz. Direct effect sensitivity on aluminum substrate was about a factor of five greater. Analysis of effects of substrate's thermal and elastic constraint disclosed insufficient effect to account for the observed increase of sensitivity. Flexoelectric effects were considered as the cause. The direct and converse sensitivity increased at approximately 2% per degree Celsius over the frequency range 0.5 to 200 Hz.

Chiral composites and lattices were studied with the aim of control and enhancement of piezoelectric properties. To that end, third-rank piezoelectricity was found to be possible in isotropic chiral solids. The highest symmetry in which piezoelectricity was thought to occur is cubic. Here, it is shown that third rank piezoelectricity can occur in isotropic chiral solids. Polarization is coupled via an isotropic third rank tensor to the antisymmetric part of the stress. Asymmetric stress can occur if balanced by moments distributed over area or volume. Such moments occur in heterogeneous solids, in which there exists a characteristic length associated with the microstructure: the Cosserat or micropolar solids. Effects associated with nonzero structure size are predicted, including radial polarization in response to torsion. These effects do not occur in gradient type flexoelectric materials; they are governed by a different tensorial rank and symmetry.

In a related vein static and dynamic effects of chirality was studied in dielectric media. Chiral dielectrics are considered from the perspective of continuum representations of spatial heterogeneity. Static effects in isotropic chiral dielectrics are predicted, provided the electric field has nonzero third spatial derivatives. The effects are compared with static chiral phenomena in Cosserat elastic materials which obey generalized continuum constitutive equations. Dynamic monopole-like magnetic induction is predicted in chiral dielectric media.

These concepts were brought to bear on design of chiral lattices with unusual elastic properties and the potential for isotropic piezoelectric response. Chiral three-dimensional lattices with tunable Poisson’s ratio were developed. Chiral three-dimensional cubic lattices are developed with rigid cubical nodules and analyzed via finite element analysis. The lattices exhibit geometry dependent Poisson’s ratio that can be tuned to negative values. Poisson’s ratio tends to zero as the cubes become further apart. The lattices exhibit stretch-twist coupling. Such coupling cannot occur in a classical elastic continuum but it can occur in a chiral Cosserat solid.

Such lattices can be made elastically isotropic by design of the structure. Chiral three-dimensional isotropic lattices with negative Poisson's ratio were developed. Chiral three-dimensional isotropic cubic lattices with rigid cubical nodules and multiple deformable ribs are developed and analyzed via finite element analysis. The lattices exhibit geometry dependent Poisson’s ratio that can be tuned to negative values. Poisson’s ratio decreases from positive to negative values as the number of cells increases. Isotropy is obtained by adjustment of aspect ratio. The lattices exhibit significant size effects. Such a phenomenon cannot occur in a classical elastic continuum but it can occur in a Cosserat solid. Such lattices were embodied in polymer via 3D printing. Piezoelectric response in these lattices is possible; testing will be proposed.