61st Annual Report on Research 2016

Mechanical spectral hole-burning^[1],[2] (MSHB) provides a novel addition^[3] to conventional LAOS (large amplitude oscillatory shear) fingerprinting^[4],[5] of polymer melts and solutions and other soft matter. The MSHB itself is a development that brings to the viscoelastic domain the ideas of nonlinear dielectric spectroscopy referred to as non-resonant spectral hole burning^[6] (NSHB) and that has been interpreted^6,[7] as being due to dynamic heterogeneity in complex liquids tested near to the glass transition temperature. Because of their low dielectric constants, the viscoelastic characterization of heterogeneity in polymers is more suitable than the dielectric counterpart.

The original works on MSHB were performed using a strain controlled rheometer, which means that the "burned" holes are in modulus space. Yet the dielectric hole burning experiments are actually carried out in dielectric compliance space. Therefore, we have been investigating the possibility of using MSHB, but now in compliance space. This required adaptation of our methodology to a stress controlled instrument and purchase from the instrument manufacturer advanced software that was capable of performing the experiments. In the following we describe the results obtained in the past year.

Experimental

Materials

The polymer solution studied in this work was a polystyrene (PS)/diethyl phthalate (DEP) solution. Linear polystyrene having a M_n=980,000 g/mol and PDI=1.16 was used. A 10% PS/DEP solution was prepared by following the methods of Qin et al.^[8]

Methods

Stress controlled MSHB experiments were carried out using a rotary rheometer with a 25 mm diameter cone and plate fixture (1° cone angle, and 50 µm truncation gap). Dynamic amplitude sweep tests and creep tests were performed to determine the linear and nonlinear stress regimes at -40 ºC. The Stress Controlled MSHB tests were then performed at the same temperature with the following procedure: one cycle sinusoidal nonlinear mechanical pump stimulation was applied, followed by a linear step in stress after a waiting time. This was repeated but the linear step was applied in the opposite direction. Comparison of the differences between the two linear steps (divided by 2) with the linear response itself gives rise to "holes" that are characteristic of the hole-burning spectroscopy.

Results and Discussion

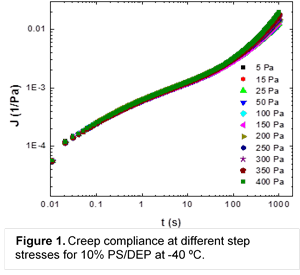
MSHB is a nonlinear viscoelastic spectroscopy that results from a comparison of the small probe response following a large sinusoidal perturbation, one first determines the linear response of the material. Figure 1 shows the linear creep response as log J(t) vs. log t where J(t) is the creep compliance and t is the lapsed creep time. Also shown in the figure are the responses for increasing stresses and, there we see that the material becomes nonlinear above approximately 50 Pa. As a result of these tests, the MSHB experiments were performed with a small stress step (after the large pump) of 25 Pa.

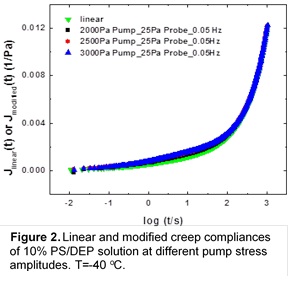
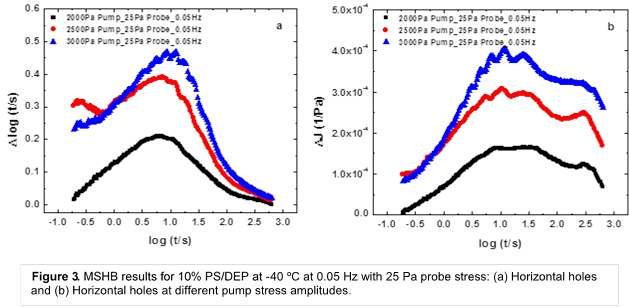
Figure 2 shows the results of a set of tests in which the pump amplitude is varied from 2000 Pa to 3000 Pa and the probe responses compared with the linear response (pump amplitude of zero). We see that in the region of approximately log t = 0 to 2 there is an increasing deviation from the linear response as the pump amplitude increases. By calculating the differences in the curves in both horizontal and vertical directions the clear horizontal and vertical holes are obtained. This is shown in Figure 3. This clear hole-burning in both horizontal (?log t) and vertical (?J(t)) directions differs from the prior results in strain controlled MSHB, where only

clear horizontal holes were observed.³ For the horizontal holes, the hole intensity increases as

the pump stress amplitude increases and slightly shifts to longer times. For the vertical holes, the intensity increases with pump amplitude but the position does not change. Despite the scatter in the data, which is also observed^6,7,[9] in NSHB dielectric tests, the main hole feature is clearly observed. Besides the first peak at around 10 s, a second peak is observed at around 300 s.

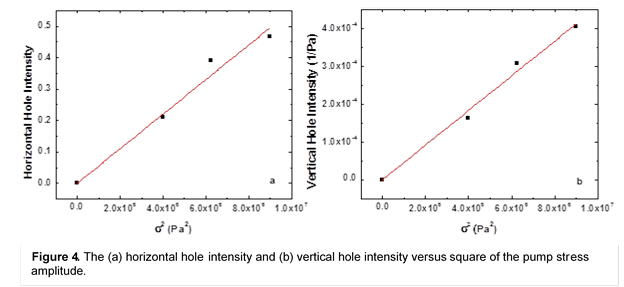
The peak intensities of the horizontal and vertical holes are plotted against the square of pump stress amplitude in Figure 4. Quadratic dependence of hole intensity on pump stress was observed for both horizontal hole and vertical hole. This relationship is consistent with the dielectric experiments^6,7,9 and our previous work^1-3,8 using strain controlled MSHB. The nonlinearity in the sample increases with the pump stress amplitude, which leads to higher hole intensity.

References

[1] X. Shi and G.B. McKenna, “Mechanical Hole Burning Spectroscopy: Evidence for Heterogeneous Dynamics in Polymer Systems,” Phys. Rev. Lett., 94, 157801-1 157801-4 (2005).

[2] X. Shi and G.B. McKenna, “Mechanical hole-burning spectroscopy: Demonstration of hole burning in the terminal relaxation regime,” Phys. Rev. B., 73, 014203-1 – 014203-11 (2006).

[3] N. Shamim and G. B. McKenna, "Mechanical spectral hole burning in polymer solutions: Comparison with large amplitude oscillatory shear fingerprinting," Journal of Rheology, 58, 43-62 (2014). http://dx.doi.org/10.1122/1.4829283.

[4] K. Hyun, M. Wilhelm, C. O. Klein, K.S. Cho, J. G. Nam, K. H. Ahn, S. J. Lee, R. H. Ewoldt, G. H. McKinley, “A review of nonlinear oscillatory shear tests: Analysis and application of large amplitude oscillatory shear (LAOS),” Progress in Polymer Science, 36, 1697–1753 (2011).

[5] G.H. McKinley, “Rheological fingerprinting of complex fluids and soft solids,” Presentation at the XVIth International Congress on Rheology, Lisbon, PT. August, 2012. Available from http://web.mit.edu/nnf/ICR2012/ICR_LAOS_McKinley_For%20Distribution.pdf.

[6] B. Schiener, R. Bohmer, A.Loidl and R.V. Chamberlin, “Nonresonant spectral hole burning in the slow dielectric response of supercooled liquids,” Science, 274, 752-754 (1996).

[7] K. R. Jeffrey, R. Richert and K. Duvvuri, “Dielectric hole burning: Signature of dielectric and thermal relaxation time heterogeneity,” J. Chem. Phys., 119, 6150-6156 (2003).

[8] Q. Qin, H. Doen and G.B. McKenna, "Mechanical Spectral Hole Burning in Polymer Solutions," J. Polym. Sci. Pol. Phys., 47, 2047-2062 (2009).

[9] B. Schiener, R.V. Chamberlin, G. Diezemann and R. Bohmer, "Nonresonant dielectric hole burning spectroscopy of supercooled liquids," J. Chem. Phys., 107, 7746-7761 (1997).