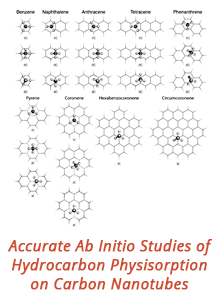
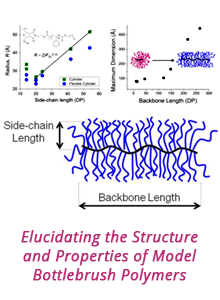
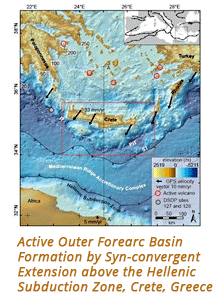
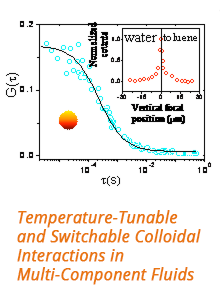
58th Annual Report on Research 2013 Under Sponsorship of the ACS Petroleum Research Fund

Our research is aimed at achieving a better understanding of the way in which electrical energy can be stored in a polymer dielectric. The basic idea underpinning the work reported here is that it should be possible to design a polymer structure that will optimize the stored energy. This involves a compromise between two conflicting criteria. In some materials, the molecules carry large permanent dipole moments, and thus interact strongly with applied fields, but polarize too easily to be able to store significant elastic energy in the distorted bonds of the molecular chain. They also suffer from having a large internal friction that leads to heat-generating losses on charging and discharging. On the other hand, some different materials are stiff enough to resist any large distortion of molecular bonds, and thus have very low dielectric losses, but show too weak a polarization to be able to store significant energy. Somewhere between these two extremes lies the optimum material.

We have introduced a simple model, and have examined how the various parameters of the model influence the amount of energy that can be stored. We have clarified a general and fundamental fact about how energy is stored in a polymer chain exposed to an electric field, namely that it resides in the potential energy due to the distortion of the molecular configuration. We have studied a detailed model in which a crystalline polymer consists of an array of parallel chains held perpendicular to an applied electric field. Permanent electric dipoles of fixed magnitude are associated with some of the individual monomers. We then suppose that each chain in this array is free to rotate about its axis without steric hindrance. This might be considered as an approximate representation of a copolymer of polyvinylidene fluoride with some larger monomers, such as chlorotrifluoroethylene, that would keep the chains apart. We assumed that the chains are sparsely crosslinked in a way that prevents rotation at the linking site. Our idealized model is then one in which chain segments of N + 1 monomers, each carrying a dipole moment, are pinned at their ends. The moments are initially parallel, and at an angle to the direction along which the electric field is to be applied. The potential energy is due to the N Hookean springs, each of spring constant K, that act between adjacent monomers. Our calculation was performed for point dipoles, and it was necessary to verify that taking into consideration the actual finite size of the molecular dipoles would not significantly alter the results. To this end, a simulation was performed, the results of which showed that the average electric field on a monomer was largely unaltered when each monomer of the PVDF was treated as a point dipole instead of a realistically sized molecule. We also investigated the Clausius-Mossotti instability, which occurs whenever the molecular polarizability is sufficiently large to cause spontaneous polarization in the absence of a field. In this situation, the polarization changes discontinuously from positive to negative as the field is reduced from positive values through zero to negative values. The stored energy is correspondingly reduced. The final results of our analysis showed that, for the particular numerical values chosen in our model, chain segments for which Nwas equal to or greater than 8 exhibited a negative apparent susceptibility at low fields. This unphysical behavior cannot occur, and so the polarization changes discontinuously, from the positive value found as the applied field approaches zero from above, to the negative of this quantity as the field is further lowered through zero from a small positive to a small negative value.

The energy density was evaluated for various N as a function of the maximum field E_b that can be applied without breakdown of the sample. For N = 2, the energy increased only slowly with E_b. As the number of monomers N per pinning site was increased, it was observed that the curvature with which the energy grows also increased, but the field at which the energy density started to saturate decreased. The optimum value of N thus decreases with increasing breakdown field. We also examined a sparse array of chains, and found that in this more complex example the ferroelectric instability occurs for N > 8, whereupon the energy density becomes negative at zero field. It is thus seen to be highly disadvantageous for energy storage if the material is in the regime where the Clausius-Mossotti transition has occurred. This requires careful choice of the number of monomers between pinning sites.