45637-AC5
Fabrication of Nano/Microstructured Organic Films using Breath Figures
Breath figures form on cold solid or liquid substrates in contact with moist air. On the liquid substrates, at low droplet coverage, nearly monodisperse size drops are able to self organize as rafts and these rafts then assemble in patterns that are similar to two-dimensional crystals full of defects. Breath figure like patterns appear on evaporating polymer solution, exposed to a blast of moist air. While on both solid and liquid substrates the latter stages in droplet growth are dominated by coalescence, in experiments done with polymer solutions, one is able to observe a highly ordered assembly of non-coalescing drops. These non-coalescing droplets eventually evaporate away leaving a film full of pores. The water droplets are usually monodisperse in size and can pack to the surface coverage approaching 0.90. In this study we elucidate the mechanism by which water drops nucleate, grow and assemble, the role played by solvent choice as well as humidity and speed of the blast of moist air and provide a theoretical framework which allows us to quantify the effect of these parameters on final assembly.
 In the past year we have performed
experiments to figure out if the model that we proposed is accurate, and we
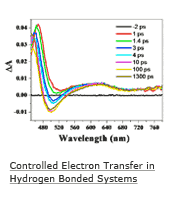
have also characterized the holey films using imaging in both real space and in
Fourier space � we describe briefly the progress made in characterizing these
films.
In the past year we have performed
experiments to figure out if the model that we proposed is accurate, and we
have also characterized the holey films using imaging in both real space and in
Fourier space � we describe briefly the progress made in characterizing these
films.
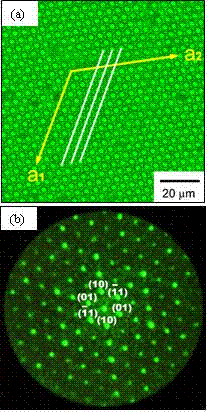
In an optical microscope, when well-collimated light is normally incident onto a sample in the transmitted mode, light will be transmitted in different directions with certain amplitude transmission function depending on the optical properties of the sample. Light waves leaving the sample film in the propagation direction are focused by an objective to a point in its back focal plane and interfere. All of the focused points form a diffraction pattern in the back focal plane with the position of each point corresponding to a diffraction angle (angle between the diffracted beam and the incident beam). For Gaussian optics (i.e., small angle or paraxial approximation), the relationship of the diffraction-point position and the sinusoid of the diffraction angle is linear. In a modern optical microscope, a series of lenses are combined to make a complex objective so that the linear approximation can be preserved out to large angles (up to the angular semi-aperture of the objective).
As shown in Fig. 1, the microporous film gives very nice, neat spot diffraction pattern with six-fold modulation, which is evident of the hexagonal order of the micropore array. Average spacing of the pores can be calculated from the diffraction pattern by two-dimensional Bragg's law, also called grating equation.� Bragg's law for transmission through a two-dimensional lattice is�
������ ml = n dhk sinq, ���������������������� (1)
where, m is the order of the diffraction maximum (m = 0, 1, 2, �), l is the wavelength of the incident light, n is the refractive index of the dispersion medium, dhk is the lattice spacing and q is the angle between the incident and diffracted beams.
A way to qualitatively check the sixfold order is to look at the coordination number (z) of every pore and the fraction (Pz) of the pores that exhibit a given coordination z. The coordination number (z) of a pore is the number of its nearest neighbors. For a perfect hexagonal lattice, all the pores should have six neighbors and P6 should be one.
In order to determine which pores are neighbors, we use a method based on Voronoi polygons. Voronoi polygon is defined as the smallest convex polygon surrounding a point whose sides are the bisectors of the lines between the point and its neighbors.
Further, the degree of order in a system can be described in terms of the entropy of conformation, defined as S = - S Pz lnPz. The smaller the value of S is, the higher the degree of order is. �We can see, in our films, most polygons are six-sided (P6 is larger than 0.9), while only a small amount of polygons have five and seven sides, and other polygons are almost nonexistent. To further quantitatively characterize the degree of the order of our hexagonally structured microporous films, we used the bond-orientational correlation function, G6(r).� Results of these studies are detailed in a publication submitted recently to Soft Matter.
We have also used the breath figure templated structures to mimic the optics found on the wingscales of a butterfly, Papilio palinurus.
This research support has been valuable to my students and my group. Some of the work was performed by my student Matija Crne who continues to work on problems related to thin films in nature.