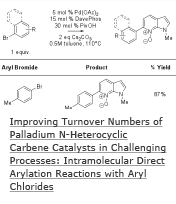
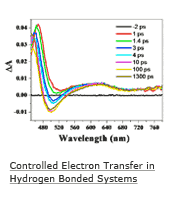
44178-AC7
Theory of Long-Range Interactions in Smectic Liquid Crystals
Some smectic liquid crystals exhibit a series of phases, including ferroelectric, antiferroelectric, and ferrielectric commensurate structures as well as one or more incommensurate phases. A long-standing problem in the physics of liquid crystals has been to understand the origin of the long-range interaction that must be present between distant layers in order for this rich variety of phases to exist. We have investigated in depth a model that incorporates thermal fluctuations in the flexing of layers, as a preliminary study has shown that it leads to a long-range interlayer interaction sufficiently strong to support a variety of commensurate and incommensurate structures. The vibrational entropy of the sequence of layers is maximized when the c-directors of all layers are parallel or antiparallel. This tendency to alignment competes with an assumed interaction between nearest-neighbor layers that favors a helical arrangement of c-directors. As a consequence, an increase in temperature leads to an unwinding of the helix that proceeds at first through commensurate phases and then into an incommensurate phase. This result is consistent with the experimentally observed "distorted clock model". It has been our goal to explore this new phenomenon in all its aspects by performing calculations on a wide variety of systems and over a wide range of material parameters. Our results should be of value both for direct comparison with experiment and as input to some existing phenomenological models. The origin of the effect that we have studied lies in the fact that the elastic constant for bending a layer of smectic-C liquid crystal along its c-director differs from the value for bending in the perpendicular direction. This gives rise to interactions between distant layers. The effect of this entropy-induced interaction is to favor a parallel or antiparallel alignment of the c-directors in these non-adjacent layers. We have calculated in detail the range and strength of this interaction in both infinite and finite samples, and find the results to depend mainly on the ratio of the average layer bending elastic constant to the layer compression modulus. At low values of this ratio, the interlayer interaction is of long range in a bulk sample, while at high values of the ratio it decays as the inverse cube of the interlayer distance. For a sample confined between rigid substrates parallel to the layers, the interaction is greatly reduced. For a free-standing film the interaction may be enhanced if the surface tension is weak, but may be diminished if the surface tension is strong. The absolute strength of the interaction that we have calculated can be expressed as a free energy per molecule that is equal to the product of the thermal energy kBT with three other factors, all of which are less than unity. Of these, the most important is an anisotropy parameter that describes the difference in elastic constants for bending a layer around an axis parallel or perpendicular to the c-director. A second factor is approximately the square of the ratio of molecular diameter to its length. The third factor is a function of the layer thickness and the ratio of the average bending elastic constant to the layer compression modulus, and has its maximum at intermediate values of this ratio. It might at first be thought that such a perturbation would be too small to modify the phase diagram. However, it turns out that the differences in free energy between the various phases of antiferroelectric liquid crystals are very small indeed. One can appreciate just how small they are by calculating the difference between the free energies of the ferroelectric and antiferroelectric phases in a typical material far from its transition temperature. We obtained this number by considering the electric field strength necessary to switch a material from its antiferroelectric phase, which has no net dipole moment per unit volume, to a ferroelectric phase having a significant dipole moment per unit volume. The free energy difference between the helical phases can be shown to be much smaller than this, and hence comparable to our calculated amount. The magnitude of the effect that we have studied is thus in the right range to have a significant effect. Nevertheless, a definitive identification of the effects of correlations in layer fluctuations may have to await realistic calculations of the interactions between adjacent layers, and this is a much more difficult task.