62nd Annual Report on Research 2017

a) Activities undertaken during the reporting period (from September 1, 2016 to August 31, 2017)

1. The first primary objective of this research project is the development of a novel and rigorous analytical solution for the borehole hydraulic fracturing initiation problem. As proposed, the new scheme for solving the general three-dimensional partial differential equations with mixed and coupled boundary conditions has been developed. The solution procedure starts with solving directly the general Biot’s poroelasticity governing equations by using the Fourier expansion theorem as well as the combined Laplace-Fourier integral transform technique. The hydraulic fracturing initiation problem is then decomposed into five fundamental, easier to handle modes based on the superposition principle. The solution procedure proposed was successfully implemented into the symbolic computational package Wolfram Mathematica, and some calculation results were obtained (Task 1 in the project schedule for isotropic saturated formation has been completed).

2. The analytical solution procedure has been further extended to cover the case of transversely isotropic rock formations. The general three-dimensional governing equations for this case is derived and represented in a compact matrix form for the convenience of solution. Parametric studies were carried out to demonstrate the impact of material anisotropy on the stress responses of the borehole and porous rock, and hence on the initiation of hydraulic fracturing. (Task 1 in the project schedule for transversely isotropic saturated formation has been completed)

3. The research group has conducted some preliminary numerical simulations for a specific situation of the targeted hydraulic fracturing initiation problem by using the commercial finite element program ABAQUS, and provided rough comparisons with the analytical solution (Task 2 in the project schedule, part of which has been completed).

b) Data supporting the outcomes of these activities

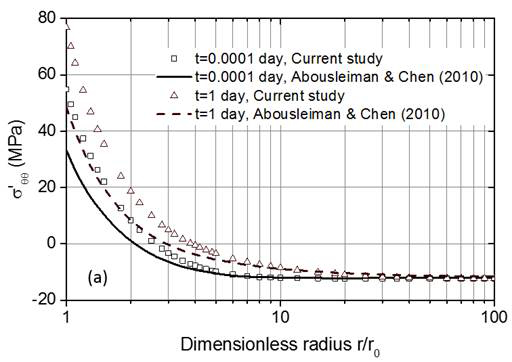
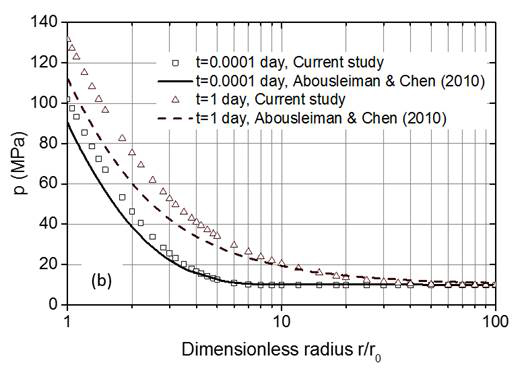
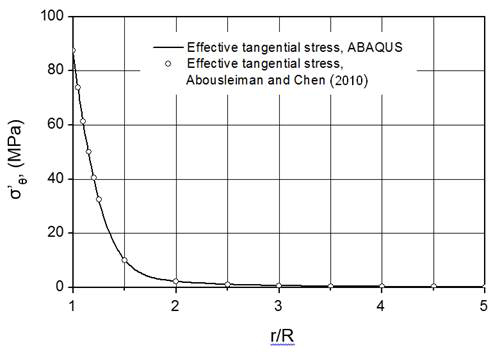
1. Fig. 1 shows the distributions of effective tangential stress and pore pressure around an inclined wellbore subjected to three-dimensional in-situ stresses and finite fluid discharge over the borehole surface. The calculated results are presented for both the currently developed rigorous method and an approximate one reported by Abousleiman and Chen (2010). It can be seen that the approximate solution profoundly underestimates the effective tangential stress induced by the fluid discharge. This indicates that the existing approximate approach for the hydraulic fracturing initiation analysis may considerably overestimate the required injection rate for triggering the fracturing.

2. Fig. 2 corresponds to the transversely isotropic case, which shows the influences of the varying material anisotropy on the calculated distribution of effective tangential stress. It is found that the anisotropy effect of the shear modulus ratio is much more significant in comparison with the Young’s modulus ratio.

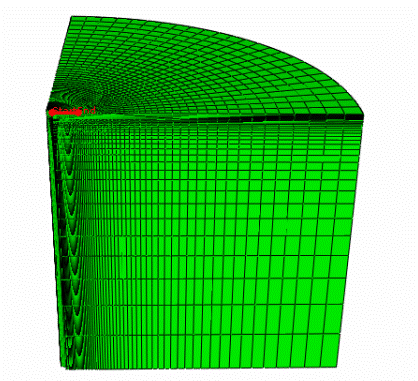
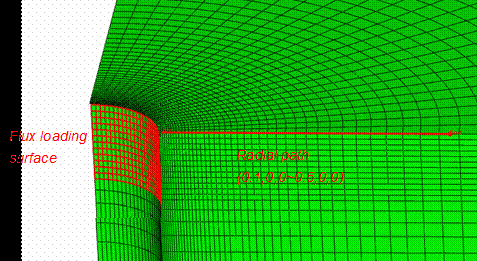
3. An ABAQUS numerical model has been established for the purely fluid flux problem, a degenerated case with the rock formation being initially unstressed. Fig. 3 shows the three-dimensional finite element meshing utilized for such specific analysis of the hydraulic fracturing initiation. And the numerical results are presented in Fig. 4 again for the effective tangential stress, which are in excellent agreement with the analytical predictions (Abousleiman and Chen, 2010). Note that to validate the reliability and meshing of the ABAQUS model, the flux rate boundary conditions at the borehole surface has been intentionally modified in the numerical simulations, so as to match exactly with those used for the approximate analytical solution. Our next step will be developing the desired user subroutines DLOAD and USDFLD for the true and correct injection boundary conditions, and then implement them into the validated ABAQUS model.

c) Impact of the research

1. The research is expected to advance our understanding on the initiation mechanism of wellbore hydraulic fracturing through analytical method and related computational modelling. The rigorous analytical solutions already developed, for both isotropic and transversely isotropic rock formations, are the first comprehensive, definite ones of their kind. These analytical solutions shall also provide a powerful hydraulic fracturing analysis/design tool for the petroleum production industry. The success of the present study on the identification of initial location and orientation of the nucleated crack will allow the PI to investigate further the hydraulic fracturing extension in the porous formations with the appropriate account of the rock toughness and its impact on the fracture propagation path. An important outcome of the research has been the two manuscripts on the analytical solution derivations: (a) Chen, S. L. (2017). Exact analytical solution for three-dimensional poroelastic responses of inclined borehole subjected to fluid injection and in-situ stresses. To be submitted to Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences; and (b) Huang, C., and Chen, S. L. (2017). Analysis of hydraulic fracturing initiation from an inclined borehole drilled in transversely isotropic poroelastic rock formation, to be submitted to International Journal of Solids and Structures. This will help the PI to build a good reputation in his research area of petroleum geomechanics and become more nationally competitive.

2. The Ph.D. and M.S. students participating in this project have been receiving a well-balanced training and research experience in the cross-disciplinary of geomechanics and petroleum engineering. Mr. Chang Huang has learned the classic integral transform method for solving the partial differential equations and the new decomposition scheme to obtain the exact analytical solution for the poromechanical responses of a borehole subjected to fluid discharge loading. Mr. Kai Liu has received the basic training in mathematics, geomechanics, and finite element modelling. He is now well equipped to move forward with the numerical simulations of this research project. Mr. Krishna Adhikari joined our group in July of 2017. He has started working towards his M.S. degree on the hydraulic fracturing initiation simulator development based on MATLAB platform (Task 3 in the project schedule currently being conducted).

Fig. 1 Variations of (a) effective tangential stress; (b) pore pressure with the normalized radial distance

Fig. 2 Influencs of (a) Young’s modulus ratio; (b) shear modulus ratio on effective tangential stress

Fig. 3 ABAQUS finite element mesh

Fig. 4 Comparison of effective radial stress between ABAQUS numerical results and approxmiate analytical solution