62nd Annual Report on Research 2017

(1)

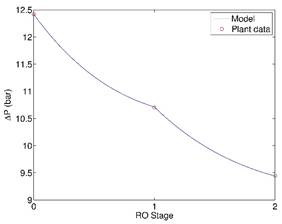
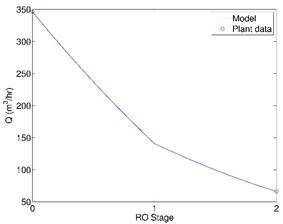
Using the least squares regression approach, the parameters in Equation (1) are determined to be  = 2.79 lmh/bar, k₂ =  1.75×10^-4 bar/(m³/h)^1.67  (in the first stage), and k₃ = 2.27×10^-2 (m³/h)^0.6/m² (in the first stage) in order to fit the measurements under normal production conditions in a RO plant in Southern California. The results are shown in Fig. 1.

(a)(b)

Fig. 1. Fitting of model parameters using plant data (a) transmembrane pressure and (b) flow rate.

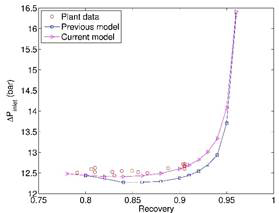
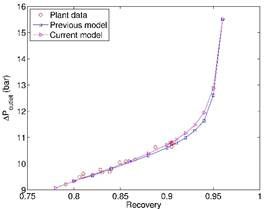
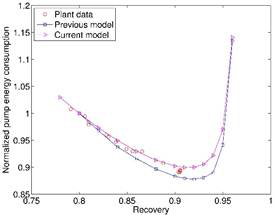
Based on the derived parameters, Equation (1) is solved under various intake flows and recoveries while maintaining the same permeate rate. The modeling results are compared with plant trial data as well as those based on an empirical model published in Desalination in 2012. While both models provide consistent and accurate prediction of transmembrane pressure at the outlet of the RO unit, Equation (1) is a little better than the previous model in predicting pump pressure and the resulting energy consumption. It matches very well with plant trial data in which the pump head is roughly constant when water recovery varies in the range of 78-90% while permeate rate is kept constant.

  (a)(b)(c)

Fig. 2. Comparison of current one-dimensional model incorporating CFD results (Eq. 1) with previously published empirical model in Desalination (2012) and measurements in a two-stage RO with a fixed permeate target. (a) transmembrane pressure at RO inlet, (b) transmembrane pressure at RO outlet, (c) normalized pump energy consumption.

The results imply that the effect of concentration polarization may not be very important in system-level modeling of large-scale industrial brackish water and wastewater RO systems where feed salinity is low. Large flows used in these systems greatly suppress the effect of concentration polarization, at the expense of a high pressure drop. Moreover, these systems are typically designed to operate far from thermodynamic equilibrium, or the transmembrane hydraulic pressure is much larger than osmotic pressure. Because the membrane length used in industrial RO desalination is much longer than the one typically seen in labs, it is believed that the longitudinal variation in salt concentration is more important than the one in the transverse direction. Therefore, Equation (1) might serve as a good complement to concentration polarization models used in literature for predicting and optimizing RO process performance in an industrial setting.

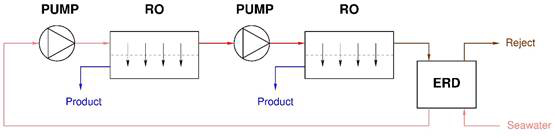
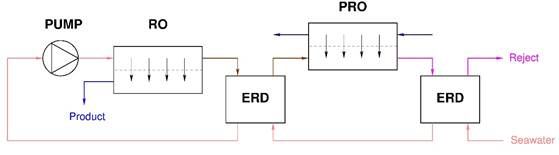
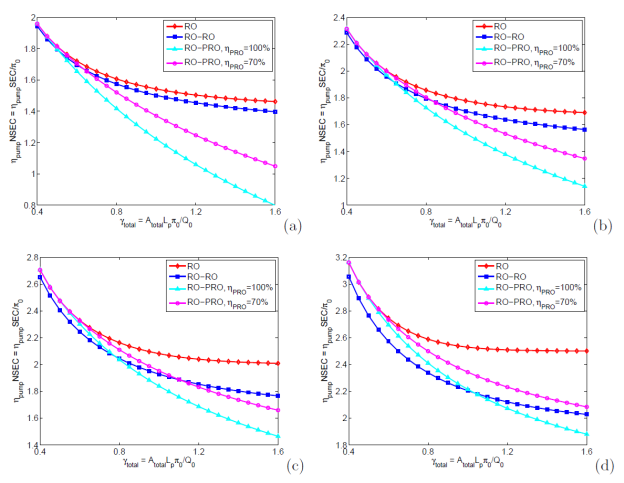
Optimization models were formulated and solved for both RO-RO and RO-PRO design configurations shown in Fig. 3. The results of Specific Energy Consumption normalized by feed osmotic pressure (NSEC) are presented in Fig. 4.

(a)

(b)

Fig. 3. Schematics of (a) RO-RO-ERD and (b) RO-PRO-ERD.

Fig. 4. Comparison of (NSEC) at different water recoveries (a) 30%, (b) 40%, (c) 50%, and (d) 60%.

The results are summarized below. Both staged RO and RO-PRO configurations reduce SEC in seawater RO desalination, provided that the membrane area is sufficient. The latter configuration is preferred when water recovery is small, a vast amount of membrane area is available, and the detrimental effects (i.e. internal concentration polarization, reverse salt leakage) in PRO are not severe. Different from hydraulic energy recovery by ERD, which has a very high efficiency, osmotic energy recovery in RO brine by PRO can only be partially done in a RO-PRO. Moreover, its amount is by no means close to the hydraulic energy recovered by ERD due to the competition of areas between the RO and PRO units. A high water recovery in RO-PRO enhances the driving force in PRO, but reduces the feed rate at the same time, limiting the volume gain ratio on the system level. It is expected that both methods will co-exist in the near future until cheaper and better membranes become available.