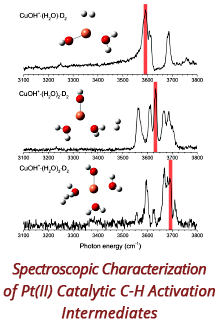
Reports: DNI952031-DNI9: Theoretical Study of the Rheology and Characteristic Length Scales of Granular Flows and Suspensions Based on an Analogy with the Plasticity of Glasses
Matthieu Wyart, PhD, New York University
Under funding from this grant we have
achieved major achievements in understanding the plasticity of amorphous solids,
granular flows and the glass transition. This work has led to publications in
peered-review journals: two manuscripts were published in Proceedings of the
National Academy of Sciences,
two in Physical Review letters, two in Soft Matter, one in
Our two main achievements are as follows:
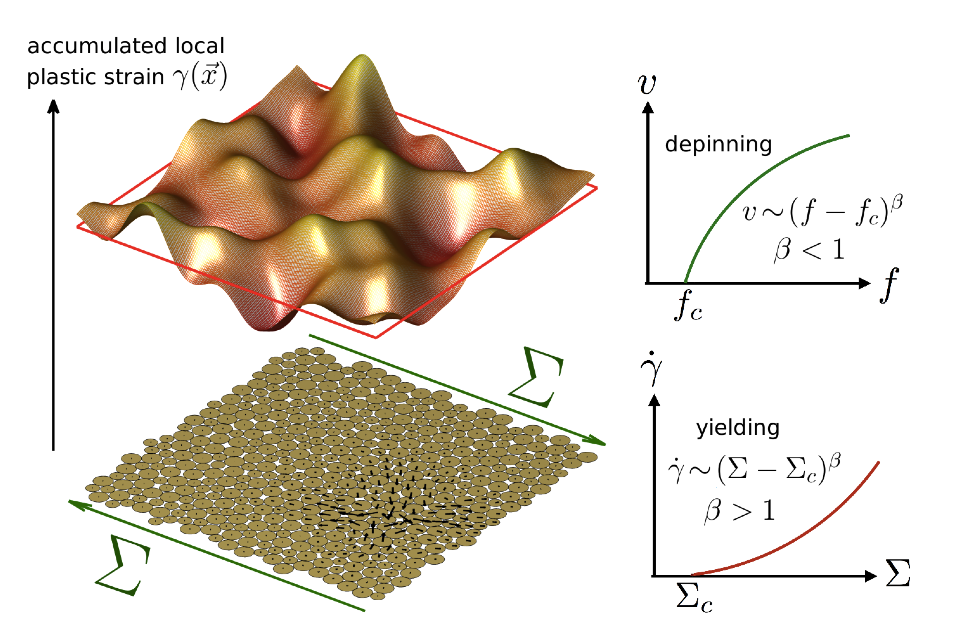
Analogy between the plasticity of amorphous solids and the depinning transition of an elastic manifold in a disordered environment.
Elasto-plasticity of soft amorphous solids:
Left:
Rheology of dense non-Brownian
suspensions: rheological properties
of dilute suspensions are well known since the early works of Einstein and Batchelor.
Their behavior in the dense limit remains mystifying however, and of critical
importance for the industry: it is central to the manufacture of materials and
their applications, and to the understanding of slurries and oil sand. As the
packing fraction of particles increases, particle motion becomes more
collective, leading to a growing length scale and scaling properties in the
rheology as the material approaches the jamming transition. There was a
knowledge gap on this question, and no convincing microscopic description of
this phenomenon. One the other hand, in recent years it had been understood
that the elasticity of simple amorphous solids was governed by a critical
point, the unjamming transition where the pressure vanishes, and where elastic
properties display scaling and a diverging length scale. The correspondence
between these two transitions was however unclear. Under ACS-PRF funding we
established a formal analogy between the rheology of the flow and the
elasticity of simple networks. Recently we used this analogy to compute the
length scales that characterize the dynamics in dense suspensions, thus solving
a long-standing debate in the field. We found that the length scale in the
fluid phase is related to the length scale that appears in certain elastic
properties of the solid phase, which diverges near the unjamming transition
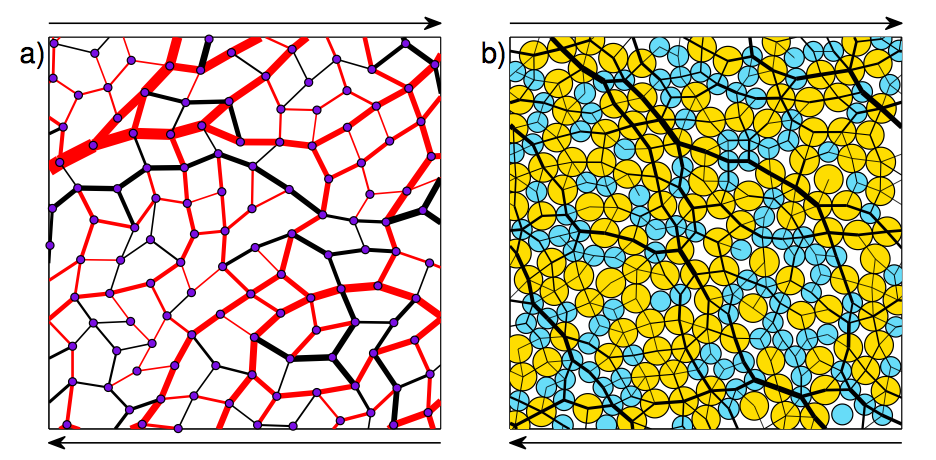
where flow starts. Fig.1 illustrates this analogy: one observes the
contact force network generated between moving particles in flow, which is very
similar to force chains observed in elastic network as shown on the left. This
analogy, together with analytical technics to study the elasticity of network,
led to these new conclusions.