59th Annual Report on Research 2014

The main objectives of this grant were to explore coherent energy transport in polymers, from theory, using both exact numerics and perturbation theories. We have made significant progress in advancing fundamental techniques and understanding on this problem using both. This grant trained one postdoctoral researcher in relaxation theory and numerical renormalization group methods, and allowed my group to explore research areas and take risks that we have not been able to do without support from the PRF. We are grateful for this support.

Energy transfer in molecular polymers is important but certain aspects are poorly understood. In particular, molecular vibrations can couple to electronic chromophores and generate energy transfer scenarios outside the scope of Foerster/Dexter and Redfield theories. These scenarios are poorly understood, but can be of immense practical and fundamental importance.

In the initial phases of this project, we focused on a donor-acceptor vibronic energy transfer system as a model for intramolecular and intramolecular energy transport in polymers. We are using numerical methods to explore energy transfer in regions where the intrasite coupling is similar to the intersite coupling. Crucially, we are exploring the role of correlated vibrational motions, strongly coupled to the electronic degrees of freedom.

Figure 1: Results for numerically exact numerical renormalization group (NRG) calculations for the population time correlation function in a two level system linearly coupled to an Ohmic harmonic bath. The quantum phase transition in this model occurs at a coupling strength of a = 1.0. These results reproduce those reported in Ref. ¹

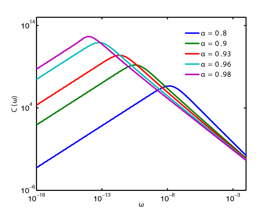
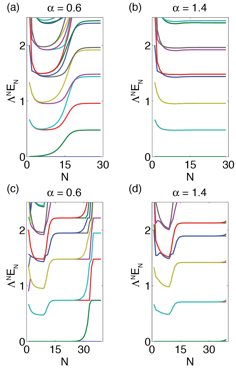
We began our investigations using the numerical renormalization group (Fig. 1 and 2). While, initially, these results appeared promising, we found significant fundamental shortcomings with the method when applied to multilevel systems and to systems with vibrational degrees of freedom. The problems with these methods appear to be rooted in the structure of the many-body eigenvalue problem when several system degrees of freedom (more than two) are included. The numerical renormalization group frames the quantum relaxation problem as a many-body problem, and iteratively diagonalizes a large matrix to find the eigenvalues of the many-body Hamiltonian. When the system includes either several energy levels or correlated vibrational motions, the numerical fixed points are nontrivial, and the flow after several iterations of the method becomes unstable (Fig. 2). Various methods, such as z-averaging, do not seem to help.Had this method worked, we would be well-positioned to comment on the degree of quantum entanglement between the electronic, vibrational, and bath degrees of freedom.

Figure 2: NRG flows for a multilevel vibronic system. NRG works by iteratively solving the many-body wavefunction of the system and bath. As the number of iterations, N, increases, the weighted energy levels should converge uniformly to "fixed points," which correspond to the many-body energies (a). As the coupling strength increases, the flow becomes unstable as N increases (c and d). We think this arises because the greater the number of system states, the smaller the energy splittings between them are. When several states come close together in energy, the NRG yields unreliable results for both statics and dynamics.

For the time being, we have tabled methods based on the NRG and instead settled on theories based on the reduced density matrix. This makes phrasing and addressing questions of entanglement more difficult. From a practical perspective, however, second-order time-dependent perturbation theories appear to be are semiquantitative when compared to exact numerical methods for model vibronic systems. We think that improvements to the analytical theories may be possible, and for the second phase of this project we are exploring those methods. We are currently drafting a manuscript that summarizes these findings, and what we think is important for vibrationally-assisted energy transfer. For the second phase of the project, we will explore molecular polymers using both molecular dynamics simulations and solutions to empirical model Hamiltonians.

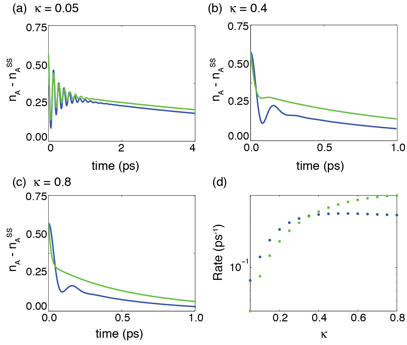
Figure 3: Hierarchical equation of motion (HEOM) approach to quantum dynamics for a multilevel system. These are the results for the population on the acceptor relative to its Boltzmann steady state for a two-level electronic system coupled to high frequency correlated intramolecular vibrations. The bath is the same as that appearin the captions of Figs. 1 and 2. Provided the Kubo parameter, i.e. the product of the bath correlation time and a, Redfield perturbation theory works semiquantitatively. Panel d shows the decay rate, extracted from an exponential fit to the time-dependent dynamics. The deviations between the Redfield theory (green dots) and the HEOM results (blue dots) are most noticeable when the Kubo parameter is greater than about a half.

1. Bulla, R.; Lee, H.-J.; Tong, N.-H.; Vojta, M., Numerical renormalization group for quantum impurities in a bosonic bath. Physical Review B 2005, 71 (4), 045122.