Reports: UR551889-UR5: Fundamental Simulations of Jamming in Confining Geometries: Effects from Surfaces, Obstacles and Exits
Amy L. Graves, PhD, Swarthmore College
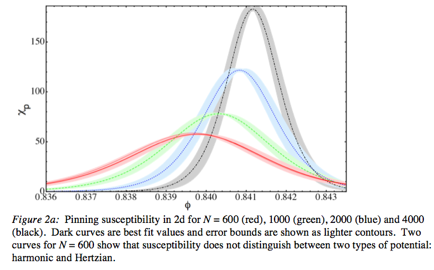
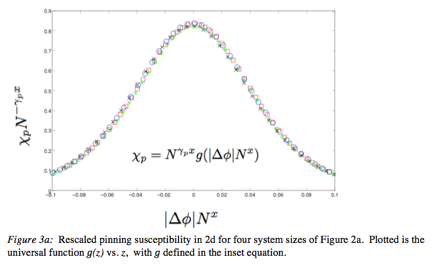
Amy L. Graves, PhD, Swarthmore College


Copyright © 2025 American Chemical Society