57th Annual Report on Research 2012 Under Sponsorship of the ACS Petroleum Research Fund
Reports: DNI651521-DNI6: Testing Models of Multi-Component Diffusion and Spin Relaxation in Porous Media under Radio Frequency Excitation in NMR
Jamie D. Walls, PhD, University of Miami
Our current research has begun to characterize the diffusion and aggregation processes (AIM IIA) for a "simple" dye, sunset yellow. We have also developed algorithms to efficiently search for relaxation selective pulses (AIM IIB), which can be used to constrain aggregation models used to model the data from AIM IIA.
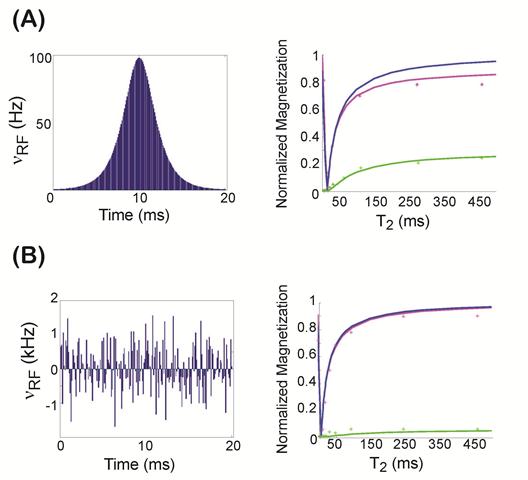
| Fig. 1. (A) Initial 20ms Soliton Pulse (left) along (right) with the magnetization profile after application of the soliton pulse vs. T2 (ms). The theoretical z (purple) and transverse (green) magnetizations, and Mtarget (blue, T2sel=15 ms) profiles are plotted (right) along with the experimental results (*). There was significant deviation of M from Mtarget. (B) The optimized pulse found from our algorithms. The magnetization profile from the optimized pulse was close to Mtarget.
|

Future Directions: Our group will employ the current pulse sequence algorithms to find pulses that are T2 selective while being robust to T1 (i.e., independent of a). We will also incorporate diffusion into our algorithms along in order to determine the necessary time-dependent pulsed field gradients that can be employed to selectively zero magnetization based not only upon T1 and T2 but also upon the value of the diffusion coefficients.
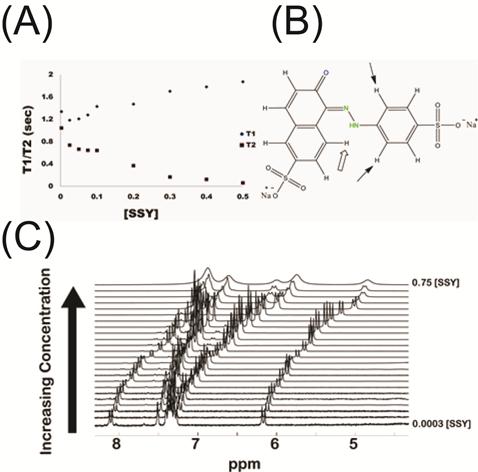
| Figure 2. (A) A plot of T1/T2 vs. concentration for sunset yellow. T1 (measured using inversion recovery experiment) increased with increasing concentration while T2 decreased (measured using the PROJECT pulse sequence). (B)The structure of sunset yellow. (C) Change in 1H NMR spectra of sunset yellow as a function of concentration from 0.01% wt to 33% wt. As the sunset yellow concentration increased, the resonances shifted up-field due increasing size of the average aggregate. |