Back to Table of Contents

46185-AC5
Void Propagation in Foam Moving in Narrow Channels

Sascha Hilgenfeldt, University of Illinois (Urbana-Champaign)

The present project studies the properties of aqueous liquid foams, i.e., dense suspensions of air bubbles in liquids, which – when interpreted as an effective medium - display non-Newtonian, viscoleastic behavior. This behavior can also be interpreted as a consequence of the microstructure, i.e., the bubble arrangement and geometry of the foam. This is crucial when studying failure (cracks) in the structure, where a void is propagating through the foam. We accomplish the void propagation by applying a well-defined driving pressure to one end of a rectangular channel containing one layer of bubbles (quasi-two-dimensional foam) with well-ordered, monodisperse bubbles.

In the project proposal, we highlighted the need to study (i) the transition between ductile, finger-like void propagation and brittle, cleavage-like void propagation; (ii) the width and possible branching of the propagating voids or cracks; and (iii) the effect of microscopic disorder (defects) on the void propagation. We have made progress in all three areas. Our experimental methods relied on taking high-speed movies and analyzing the resulting images.

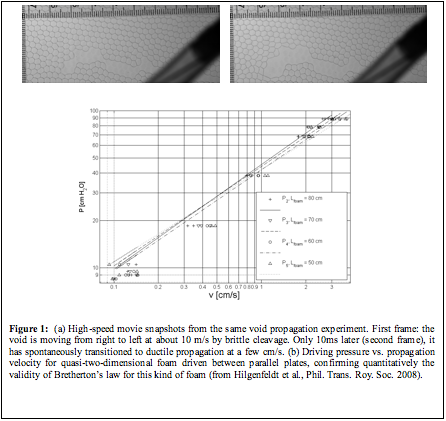
The transition between the two states of void propagation was found to occur in our experiments not just as a function of external parameters (driving pressure or applied rate of stress), but spontaneously during the void propagation, and then always from brittle to ductile (Fig.1a). As ductile cracks always propagate at dramatically lower velocities than brittle cracks, the question arises if there is a critical velocity for ductile crack propagation. We were able to answer this question using a classical Bretherton experiment, measuring the capillary number of bubble motion as a function of driving pressure (Fig. 1b). This established a direct relation between local velocity of bubble motion and driving. Employing a fluid-mechanical force balance, we could show that above a critical velocity (which depends only on bubble geometry and foam liquid content), ductile propagation becomes impossible as films elongate and break (Hilgenfeldt, S. Arif, and J.-C. Tsai, Phil. Trans. Roy. Soc. 2008). Our experiments confirm that ductile cracks always propagate below this critical velocity and brittle cracks are always faster (see also: S. Arif, Presentation at the 2007 APS Division of Fluid Dynamics meeting).

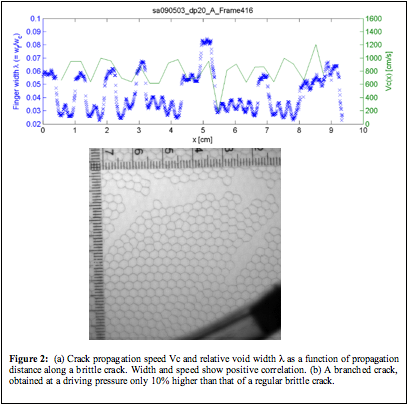
We found that, in the case of brittle propagation, not only do the width of the crack and its speed depend on the driving pressure, but also the morphology of the void, including irregularities and branching. We found clear positive correlations between crack speed and relative crack width for controlled brittle propagation (see Fig.2a), and a rapid increase in crack speed and irregularity with increased driving pressure, including branching (see Fig.2b). The latter are both well-known phenomena in crack propagation in traditional hard solids (particularly metals) and can here be studied easily in detail on the scale of single "atoms" (bubbles). The dependence of speed on relative crack width (evaluated as a fraction of the width of the confining channel) indicates that confinement of such cracks serves to slow them down.

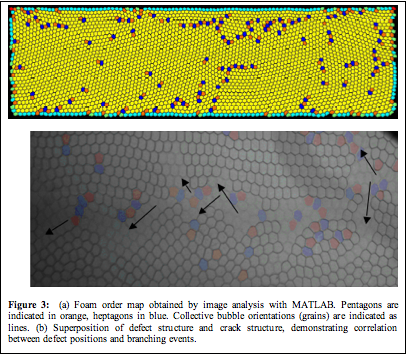
Irregularities and branching in crack propagation, as well as the precise moment of transition from brittle to ductile propagation, are all correlated with the position of frozen defects in the foam, typically those of 5-sided and 7-sided bubbles (penta/hepta defects). We have developed MATLAB routines to automatically detect and classify these defects (see Fig.3a as an example). These structures represent a quantifiable change in the background stress field in the material, and their positions are strongly correlated with changes in crack direction and attempted branching events (see Fig.3b). We have worked on quantifying the stress field and determining under what circumstances the crack will be deflected, branched, or arrested in its brittle propagation by the presence of a defect (S. Arif, Presentation at the 2008 APS Division of Fluid Dynamics meeting).

Back to top