ACS PRF | ACS
All e-Annual Reports

41945-AC10
Noise Spectroscopy of Spin-Based and Semiconductor-Based Magnetoresistive Systems
The number of applications for magnetic sensors has grown explosively in the past two decades.� In particular, the demand for small, low-power magnetic sensors has grown exponentially.� Applications abound to meet the needs of users in the medical, military, information technology, and industrial communities.� However, one area in which little progress has been made in recent years is small, inexpensive, low-power, low frequency sensors capable of detecting ultra-low magnetic fields.� The detection of fields between a femtoTesla (10-11 Oe)� to about 100 picoTesla (10-6 Oe) has been dominated by relative large, expensive, power-hungry sensors such as� fluxgates, optically pumped magnetometers, and SQUIDs.� If significant progress could be made in producing small, inexpensive, low-power magnetic sensors the technological impact would be great.�
The most likely technology to make such progress is magnetic-tunnel-junction (MTJ) sensors combined with frequency-modulated magnetic flux concentrators to suppress 1/f noise.� The recent developments that make this approach so attractive are the achievement of well over 100% values of the tunneling magnetoresistance (TMR) in MTJs, the demonstration of magnetic thin films with a saturation field of 5x10-6 Tesla, and the development magnetic flux concentrators that can operate at 104 Hz.�� To evaluate the combined effect of these properties, we have derived a theoretical model and incorporated it in a spreadsheet for easy evaluation of the expected performance of the sensors.� The model is based on our experimental studies of noise in MTJ sensors and uses the empirical parameters for electronic and magnetic 1/f� noise contributions that were obtained from our experiments.
The equation governing the minimum detectable field for a Wheatstone bridge of MTJ sensors is the following.
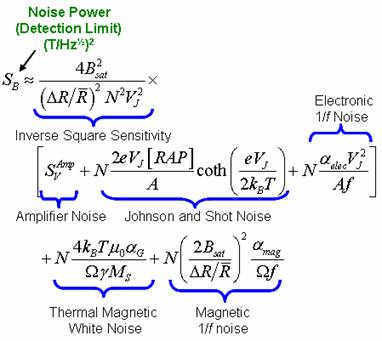 Eq. 1
Eq. 1
The parameters in Eq. 1 are as follows. SB: sensor noise power, T2/Hz; Bsat: saturation field of free layer, T; ΔR: resistance change of one MTJ from parallel to antiparallel magnetization; R: resistance of MTJ in orthogonal magnetization state; N: number of MTJ� in each leg of the Wheatstone bridge; VJ: voltage drop across each MTJ; SvAmp : amplifier voltage noise power; e: electronic charge; RAP: resistance area product of each MTJ; A: area of each MTJ; kB: Boltzmann's constant; T : absolute temperature; αelec: electronic 1/f Hooge parameter; f : frequency of operation of MEMS flux concentrator; mo: permeability of free space; αG: Gilbert damping parameter; W: free-layer volume in each MTJ; g: gyromagnetic ratio for an electron; Ms: saturation magnetization of the free layer; αmag: magnetic 1/f Hooge parameter.
Equation 1 has been incorporated in a spreadsheet to enable rapid evaluation of the effect of changing the various sensor parameters.� The evaluation enables rapid optimization of sensor performance.� One of the most valuable aspects of the evaluation process is that it allows the user to avoid over-design in achieving some of the more difficult sensor goals.� For example, it was found that the level of magnetic 1/f noise meant that TMR values in excess of 100% were so far into the regime of diminishing return as to be pointless.
The major conclusions of this work may be summarized as follows: 1) Recent advances in TMR, free-layer saturation field, and MEMS oscillating flux concentrators suggest that it may be possible to extend small, inexpensive, low-power, ultra-sensitive magnetic sensors; 2) The major challenge is to integrate these advances into sensors; 3) Our modeling has shown that there exists an unexpected new regime for magnetic sensors in which increasing the number of MTJs reduces the sensor performance.� Many current designs seem to lie in this regime; 4) If successful, these sensors will play important roles in a wide range of applications including healthcare, homeland security, and national defense.� Current work focuses on gaining fundamental understanding of quasi-thermal 1/f magnetization fluctuations, specifically, the extent to which these fluctuations are due to hysteresis.