www.acsprf.org

1. Introduction

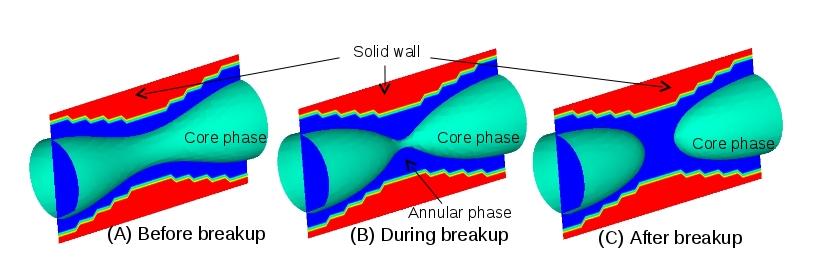
Water-lubricated transport is an attractive technology for delivery of crude oils in pipelines. In this technology, water is used to enclose the oil in a pipe as a sheath, and the resulting core-annular flow provides a simple mechanism to reduce a high pressure required to pump the oil. However, fragmentation of the oil-water interface may occur due to the combined effect of inertia, surface tension, and surface-laden surfactants, and as a result, different flow regimes such as drops, slugs, and emulsions, can be formed, which adversely affects the effectiveness of the method.

Previous studies of core-annular flows have focused mainly on two-dimensional (2D) flow, and the fragmentation is rarely investigated. In the project, the following specific objectives have been pursued:

1. Develop an accurate numerical method capable of modeling three-dimensional (3D) two-phase flows in arbitrary geometries, where the fluid-fluid interface will be handled by the immersed-interface method and the topology change due to the fragmentation of the interface will be incorporated.
2. Validate the numerical method against previously established results involving two-fluid interfaces.
3. Study the effects of the viscosity ratio, capillary number, and Reynolds number and characterize the topology of the interface for the core-annular flow.

The original objective of modeling the two-phase flow in a straight cylindrical pipe has been extended to modeling the flow in arbitrary geometries. This extension is motivated by the fact that the real-world pipeline system often consists of non-cylindrical devices such as fittings and valves and these complex geometries have a significant effect on stability and breakup of the core-annular flow.

To handle the arbitrary shape of the pipe wall, an efficient immersed-boundary method has been developed through this project. In this method, only Cartesian grids are used to discretize the domain; the arbitrary wall geometry is handled by a second-order sharp-interface method; and the time-varying fluid-fluid interface is handled by a diffuse-interface method. Since grid generation is straightforward, the method is particularly useful to deal with complex- and moving-boundary problems often encountered in engineering.

In the current numerical method, a level-set function has been incorporated to track the liquid-liquid interface. Several tests have been performed to validate the numerical method. It has been demonstrated that the method can handle the 3D fragmentation of the core-annular flow. Finally, we apply this newly developed method to simulate the motion of drops through a non-trivial wall geometry. The fundamental effect of the viscosity ratio, capillary number, and Reynolds number has been studied. The effect of the surfactant is not considered in this project. A journal article is currently being prepared.

2. Development of the numerical method

The overall numerical approach and the separate treatments of the fluid-fluid and the fluid-solid interfaces have been described in the previous annual report of this project. In Year 2, the following improvements have been made to enhance the numerical approach:

1. A 5th-order Weighted Essentially Non-Oscillatory (WENO) scheme has been introduced into the spatial discretization of the level-set function. This scheme significantly improves the accuracy and stability of the interface tracking.
2. A commonly used mass-compensation approach has been implemented to improve the mass conservation of the numerical method.
3. A curvature smoothing technique has been introduced to suppress the numerical instability caused by oscillations in the curvature calculation for the fluid-fluid interface.
4. To implement parallel algorithm and accelerate 3D simulations, a sparse linear system solver, AZTEC, has been successfully tested in the current immersed-boundary framework for solving the momentum and pressure equations.
5. Other than the validation described in the previous report, an additional test involving evolution of the two-fluid interface in a wavy channel has been performed and has successfully validate the treatment of the solid boundary.

The capability of the current method in capturing the arbitrary wall geometry and also the topological change of the two-fluid interface is demonstrated in Figure 1.

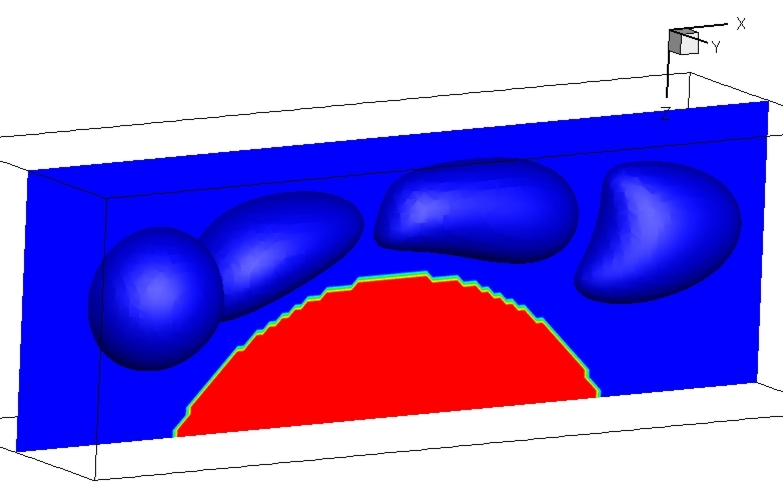
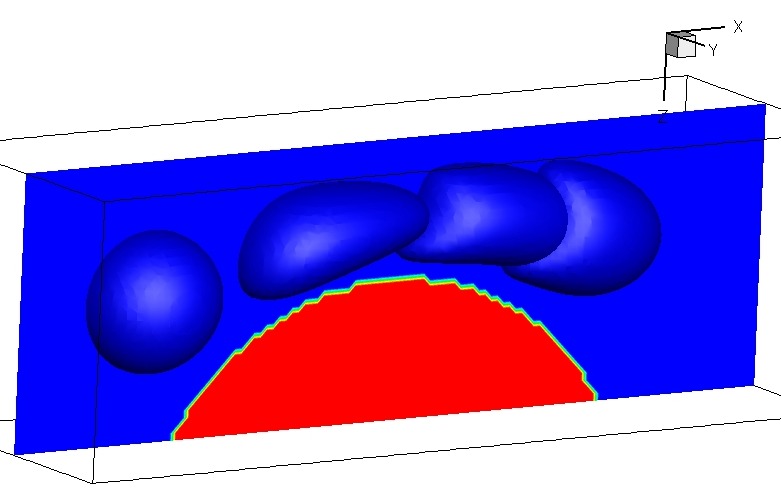
3. Study of the fragmented drops through a constricted channel

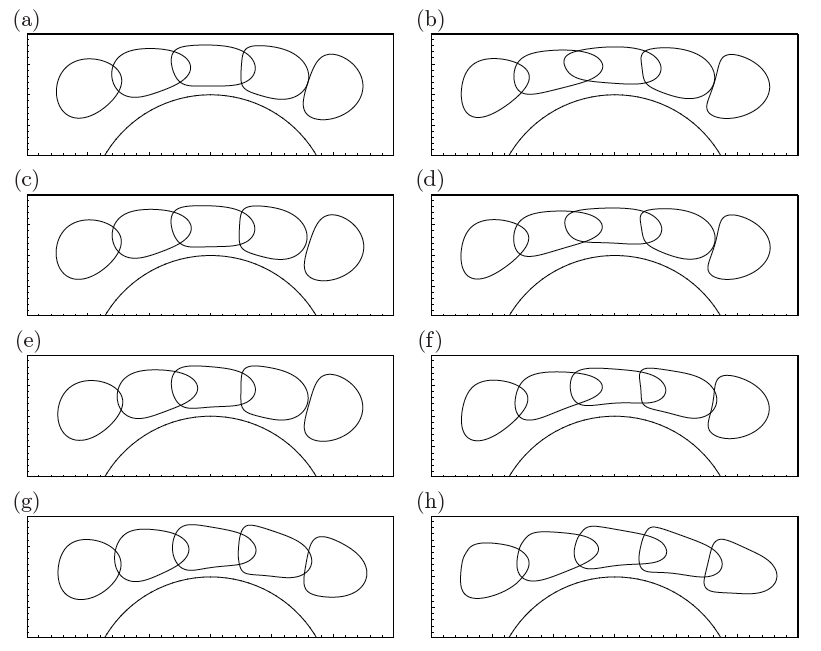
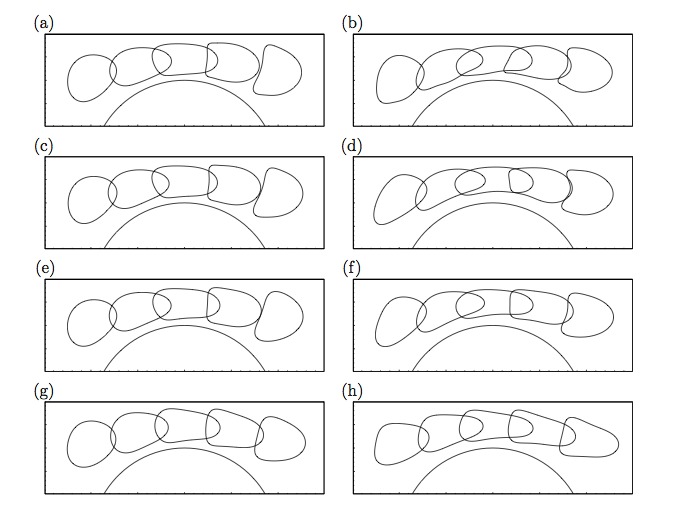
A constricted channel represents a typical case of varying cross section in pipeline transport. We considered an array of drops going through a semi-circular hurdle and systematically studied deformation of the drops under different combinations of the viscosity ratio (λ), capillary number (Ca), and Reynolds number (Re). A periodic configuration in the streamwise direction is considered.

Figures 2 and 3 show the typical shape of the 2D drop as it goes over the hurdle at different Ca, λ, and Re. The results demonstrate that all three parameters may have significant effect on the instantaneous shape of the drop.

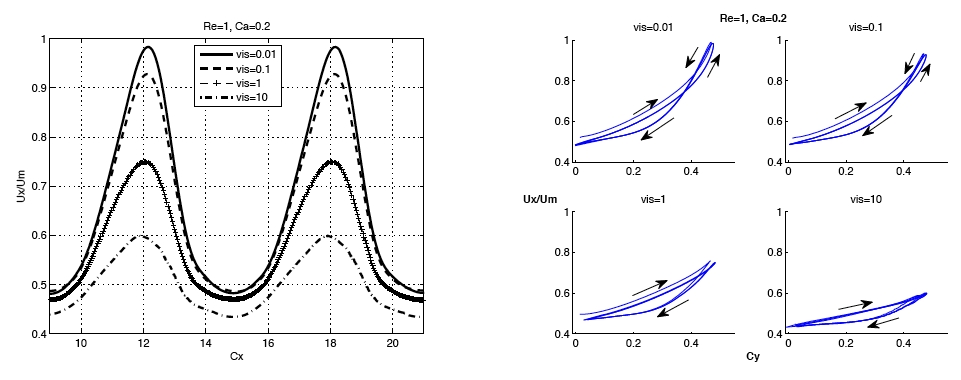
Figure 4 shows the instantaneous translational velocity of the drop over the hurdle. As λ is increased (i.e., the drop becomes more viscous), the translation of the drop is slowed down significantly, and in addition, the wall-normal migration of the drop becomes nearly linear with respect to its distance to the wall.

The corresponding 3D result is shown in Figure 5. According to our numerical simulation, the translation of the 3D drop is faster than the 2D drop under the same condition. The shape of the drop is also significantly different than the 2D drop. The further investigation of the 3D effect is ongoing.
4. Educational aspect of the project

A graduate student, Mr. Bo Yin, has received extensive training in the numerical method of multiphase flows through this grant. The physics and computational modeling of multiphase flows have been incorporated into an undergraduate/graduate course, Computational Fluid Dynamics and Multiphysics Modeling, that the PI teaches at Vanderbilt University.

---

Figure 1: Three-dimensional breakup of core-annular flow.

Figure 2: 2D drop through a constricted channel where Re=1, Ca=0.5 (left panels) and 1 (right panels), and from top to bottom, λ=0.01, 0.1, 1, and 10.

Figure 3: The same problem with Re=50.

Figure 4: Translational velocity of the drop through the constricted channel as a function of its x- and y-positions.

Figure 5: A 3D drop going through the hurdle (red) with Re=1, Ca=0.2, and λ=0.01 (upper panel) and 1 (lower panel).